Quantum Leap in Solving Nonlinear Differential Equations: A Groundbreaking Approach Using Effective Hamiltonians
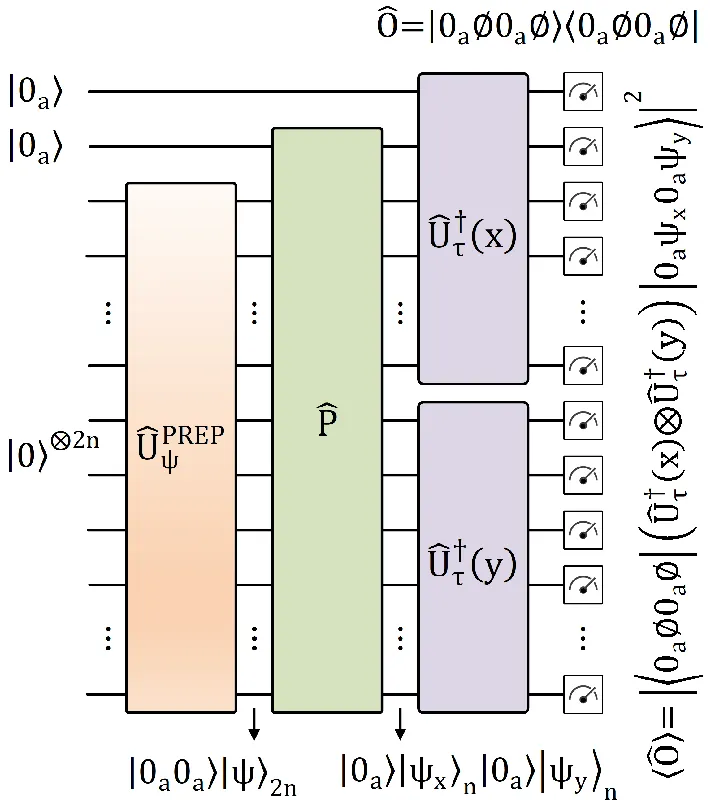
In a landmark research paper, Hsin-Yu Wu and colleagues unveil a novel quantum algorithm capable of tackling linear and nonlinear differential equations (DEs) using effective Hamiltonians. This innovative method, situated at the intersection of quantum computing and machine learning, stands to revolutionize how complex equations are solved in various scientific and engineering fields.
Revolutionizing Problem Solving with Quantum Mechanics
The core premise of this research is to leverage quantum mechanics to construct effective Hamiltonians that encapsulate the properties of DEs in a latent space. By encoding differential and data constraints into these Hamiltonians, the authors offer a pathway for efficient problem-solving without relying on traditional variational methods that can be computationally intensive and time-consuming.
Encoding the Problem: The Power of Chebyshev Polynomials
Central to the algorithm's success is the use of Chebyshev polynomials. These mathematical constructs provide a robust framework for approximating functions and solving equations. By formulating the problem within the Chebyshev space, the algorithm enables a more flexible evaluation of solutions that extends beyond traditional fixed grid points, a common limitation in numerical methods.
A Physics-Informed Approach
This algorithm not only simplifies the representation of differential equations but also integrates physics-informed constraints. This means that the solutions derived from quantum computations are guided by the physical realities of the systems being modeled, enhancing the validity of the outcomes. As a result, the research provides scientists and engineers with a powerful tool for addressing complex challenges across domains—from simulations of fluid dynamics to predictive modeling in financial analysis.
Technical Nuances Made Accessible
For those unfamiliar with the intricacies of quantum algorithms, the research simplifies complex ideas into understandable components. The effective Hamiltonian approach involves preparing the ground state of the Hamiltonian through methods such as quantum imaginary-time evolution. This technique allows researchers to obtain low-energy states that correspond to solutions of the differential equations.
Implications for the Future of Scientific Computing
The advances discussed in this paper may herald a new era for scientific computing, particularly as quantum technologies mature. As real-world applications expand, from chemical reactions to deep learning models in various scientific fields, the potential for this quantum algorithm to solve previously intractable problems becomes increasingly clear. It represents a fusion of theoretical physics and practical computation, paving the way for future innovations in quantum technologies.
In conclusion, this research not only addresses significant challenges in solving differential equations but also sets a precedent for how quantum computing can be effectively utilized in real-world scenarios. By marrying the principles of physics with advanced computational techniques, the authors provide a roadmap that could potentially reshape the landscape of scientific inquiry.