Unraveling the Dynamics of Solitons: Innovations in Coarse-Grained Space-Time Methods
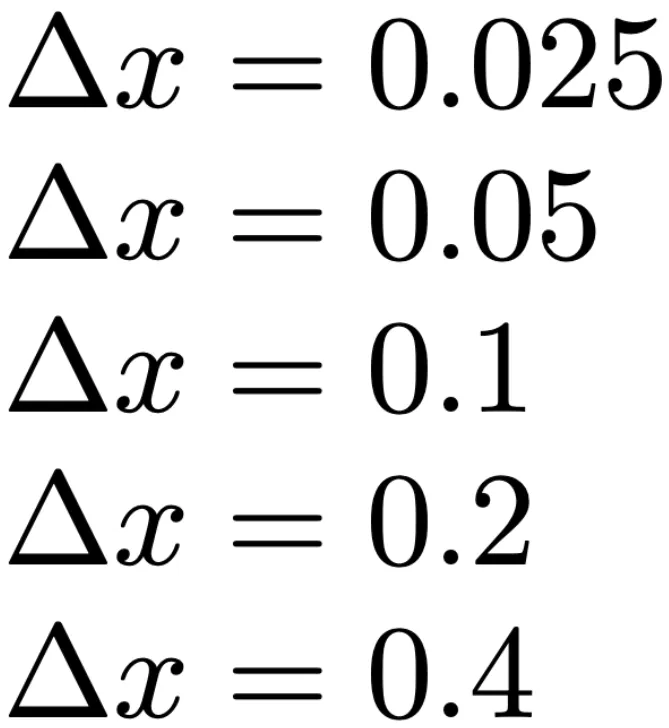
Recent research led by Dung N. Pham and his team at Princeton University has taken a significant leap in understanding the long-time behavior of solitons through a novel coarse-graining approach. Their study dives deep into the Sine-Gordon model, a cornerstone of theoretical physics, exploring its implications in real-world applications, particularly within Josephson junctions.
The Challenge of Long-Time Soliton Dynamics
The Sine-Gordon equation is essential for analyzing the formation and dynamics of solitons, which are stable, localized wave packets. One particularly challenging aspect of this equation is capturing soliton behavior over long time periods, as numerical methods can struggle under perturbations. The research team sought to address these challenges by refining a coarse-graining approach applied in space-time, which uses dual-mesh construction based on the Minkowski metric.
This method was initially validated against established models, such as the dynamics of fluxons in Josephson transmission lines (JTLs). The researchers demonstrated that their approach significantly enhances numerical stability and can sustain the integrity of soliton solutions across lengthy simulations.
Introducing "Schwinger Atoms"
A key finding of the research was the identification of long-lived bound states, termed "Schwinger atoms". These are configurations where a soliton becomes trapped around a fixed central charge, much like an electron orbiting the nucleus in an atom. Under specific conditions, the system transitions to exhibit limit cycles resembling positronium-like states, enabling an intriguing manifestation of stable interactions between solitons.
In this framework, solitons transformed into coherent structures, indicating a broader potential to simulate relativistic quantum field theories using JTLs. This emerging perspective could revolutionize our approach to quantum simulations, linking classical phenomena to quantum behaviors.
Methodological Innovations for Non-Perturbative Analysis
The incorporation of outgoing boundary conditions is another technical highlight of this research. By allowing for radiative dissipation, the study provides a framework for shifting focus from finite computational domains to those mirroring infinite ones. This aspect proves crucial in examining the long-term stability and evolution of solitons.
The researchers anticipate their spatio-temporal coarse-graining methodology could pave the way for future investigations into the non-perturbative structure formation in non-linear field theories, potentially leading to groundbreaking insights in particle physics, condensed matter systems, and beyond.
Conclusion: Implications for Future Research
Overall, the innovative approaches established in this paper highlight a promising avenue for exploring non-perturbative phases of matter and deepen our understanding of soliton dynamics. As researchers branch into more complex configurations and apply these findings to real-world materials, the implications for fields such as quantum information science and superconducting technologies could be profound.
Engaging with the dynamics of these soliton structures not only enhances scientific knowledge but could also contribute to the development of advanced technologies, further integrating fundamental physics with practical applications.